Skip to content →

Tag: puzzle

the Reddit (after)effect

Published January 15, 2011 by lievenlb

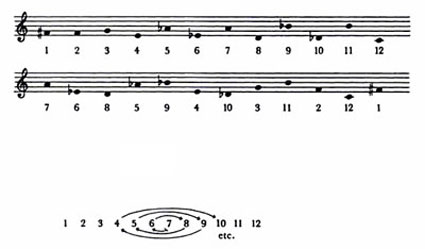
Sunday january 2nd around 18hr NeB-stats went crazy.

Referrals clarified that the post ‘What is the knot associated to a prime?’ was picked up at Reddit/math and remained nr.1 for about a day.

Now, the dust has settled, so let’s learn from the experience.

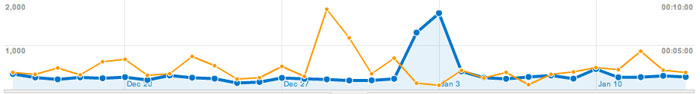
A Reddit-mention is to a blog what doping is to a sporter.

You get an immediate boost in the most competitive of all blog-stats, the number of unique vistors (blue graph), but is doesn’t result in a long-term effect, and, it may even be harmful to more essential blog-stats, such as the average time visitors spend on your site (yellow graph).

For NeB the unique vistors/day fluctuate normally around 300, but peaked to 1295 and 1733 on the ‘Reddit-days’. In contrast, the avg. time on site is normally around 3 minutes, but dropped the same days to 44 and 30 seconds!

Whereas some of the Reddits spend enough time to read the post and comment on it, the vast majority zap from one link to the next. Having monitored the Reddit/math page for two weeks, I’m convinced that post only made it because it was visually pretty good. The average Reddit/math-er is a viewer more than a reader…

So, should I go for shorter, snappier, more visual posts?

Let’s compare Reddits to those coming from the three sites giving NeB most referrals : Google search, MathOverflow and Wikipedia.

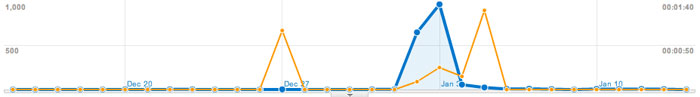
This is the traffic coming from Reddit/math, as always the blue graph are the unique visitors, the yellow graph their average time on site, blue-scales to the left, yellow-scales to the right.

Here’s the same graph for Google search. The unique visitors/day fluctuate around 50 and their average time on site about 2 minutes.

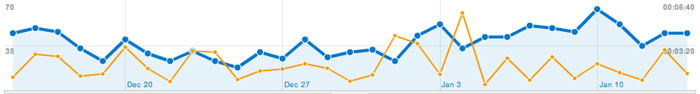
The math-related search terms most used were this month : ‘functor of point approach’, ‘profinite integers’ and ‘bost-connes sytem’.

More rewarding to me are referrals from MathOverflow.

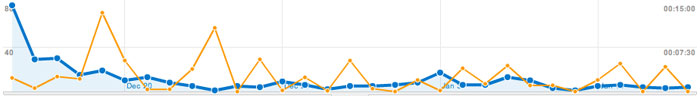
The number of visitors depends on whether the MathO-questions made it to the front-page (for example, the 80 visits on december 15, came from the What are dessins d’enfants?-topic getting an extra comment that very day, and having two references to NeB-posts : The best rejected proposal ever and Klein’s dessins d’enfant and the buckyball), but even older MathO-topics give a few referrals a day, and these people sure take their time reading the posts (+ 5 minutes).

Other MathO-topics giving referrals this month were Most intricate and most beautiful structures in mathematics (linking to Looking for F-un), What should be learned in a first serious schemes course? (linking to Mumford’s treasure map (btw. one of the most visited NeB-posts ever)), How much of scheme theory can you visualize? (linking again to Mumford’s treasure map) and Approaches to Riemann hypothesis using methods outside number theory (linking to the Bost-Connes series).

Finally, there’s Wikipedia

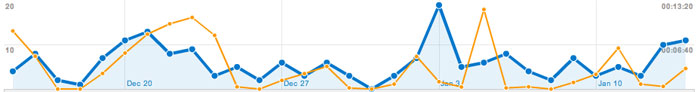
giving 5 to 10 referrals a day, with a pretty good time-on-site average (around 4 minutes, peaking to 12 minutes). It is rewarding to see NeB-posts referred to in as diverse Wikipedia-topics as ‘Fifteen puzzle’, ‘Field with one element’, ‘Evariste Galois’, ‘ADE classification’, ‘Monster group’, ‘Arithmetic topology’, ‘Dessin d’enfant’, ‘Groupoid’, ‘Belyi’s theorem’, ‘Modular group’, ‘Cubic surface’, ‘Esquisse d’un programme’, ‘N-puzzle’, ‘Shabat polynomial’ and ‘Mathieu group’.

What lesson should be learned from all this data? Should I go for shorter, snappier and more visual posts, or should I focus on the small group of visitors taking their time reading through a longer post, and don’t care about the appallingly high bounce rate the others cause?

8 Comments

Olivier Messiaen & Mathieu 12

Published December 31, 2009 by lievenlb

To mark the end of 2009 and 6 years of blogging, two musical compositions with a mathematical touch to them. I wish you all a better 2010!

Remember from last time that we identified Olivier Messiaen as the ‘Monsieur Modulo’ playing the musical organ at the Bourbaki wedding. This was based on the fact that his “modes à transposition limitée” are really about epimorphisms between modulo rings Z/12Z→Z/3Z and Z/12Z→Z/4Z.

However, Messiaen had more serious mathematical tricks up his sleeve. In two of his compositions he did discover (or at least used) one of the smaller sporadic groups, the Mathieu group $M_{12} $ of order 95040 on which we have based a whole series of Mathieu games two and a half years ago.

Messiaen’s ‘Ile de fey 2’ composition for piano (part of Quatre études de rythme (“Four studies in rhythm”), piano (1949–50)) is based on two concurrent permutations. The first is shown below, with the underlying motive rotational permutation shown.



This gives the permutation (1,7,10,2,6,4,5,9,11,12)(3,8). A second concurrent permutation is based on the permutation (1,6,9,2,7,3,5,4,8,10,11) and both of them generate the Mathieu group $M_{12} $. This can be seen by realizing the two permutations as the rotational permutations



and identifying them with the Mongean shuffles generating $M_{12} $. See for example, Dave Benson’s book “Music: A Mathematical Offering”, freely available online.

Clearly, Messiaen doesn’t use all of its 95040 permutations in his piece! Here’s how it sounds. The piece starts 2 minutes into the clip.

The second piece is “Les Yeux dans les Roues” (The Eyes in the Wheels), sixth piece from the “Livre d’Orgue” (1950/51).



According to Hauptwerk, the piece consists of a melody/theme in the pedal, accompanied by two fast-paced homorhythmic lines in the manuals. The pedal presents a sons-durées theme which is repeated six times, in different permutations. Initially it is presented in its natural form. Afterwards, it is presented alternatively picking notes from each end of the original form. Similar transformations are applied each time until the sixth, which is the retrograde of the first. The entire twelve-tone analysis (pitch only, not rhythm) of the pedal is shown below:



That is we get the following five permutations which again generate Mathieu 12 :

  • a=(2,3,5,9,8,10,6,11,4,7,12)
  • b=(1,2,4,8,9,7,11,3,6,12)(5,10)=e*a
  • c=(1,12,11,9,5,4,6,2,10,7)(3,8)=e*d
  • d=(1,11,10,8,4,5,3,7,2,9,6)
  • e=(1,12)(2,11)(3,10)(4,9)(5,8)(6,7)

Here’s the piece performed on organ :

Considering the permutations $X=d.a^{-1} $ and $Y=(a.d^2.a.d^3)^{-1} $ one obtains canonical generators of $M_{12} $, that is, generators satisfying the defining equations of this sporadic group

$X^2=Y^3=(XY)^{11}=[X,Y]^6=(XYXYXY^{-1})^6=1 $

I leave you to work out the corresponding dessin d’enfant tonight after a couple of glasses of champagne! It sure has a nice form. Once again, a better 2010!

5 Comments

When was the Bourbaki wedding?

Published October 7, 2009 by lievenlb

It’s great fun trying to decode some of the puns contained in Betti Bourbaki’s wedding invitation. Below a photograph, taken on May 13th 1939, of three of the practical jokers (from left to right : Ralph Boas, Frank Smithies and Andre Weil), the others were Claude Chabauty, Weil’s wife Eveline and Louis Bouckaert (from Louvain).



Part of this picture is on the front cover of the book Lion Hunting & other Mathematical Pursuits. This book clarifies the ‘Secrétaire de l’Oevre du Sou du Lion’-phrase as well as some of the names on the card.

Inspired by the Bourbaki-hoax, a group of postdoctoral fellows visiting Princeton University in 1937-1938 (Boas, Smithies and John Tuckey) published their inventions, allegedly devised by Hector Pétard (aka ‘H(oist) W(ith) O(wn) Petard’ after the Shakespeare line “For ’tis the sport to have the engineer, hoist with his own petard…” Hamlet act III scene IV) who was writing under the pseudonym of E.S. Pondiczery. Pétard’s existence was asserted in the paper “A Contribution to the Mathematical Theory of Big-Game Hunting” Amer. Math. Monthly 45 (1938) 446-447.

Smithies recalls the spring 1939 period in Cambridge as follows : “The climax of the academic year, as far as we were concerned, came in the Easter term. André Weil, Claude Chabauty, and Louis Bouckaert (from Louvain) were all in Cambridge, and the proposal was mooted that a marriage should be arranged between Bourbaki’s daughter Betti and Hector Pétard; the marriage announcement was duly printed in the canonical French style – on it Pétard was described as the ward of Ersatz Stanislas Pondiczery – and it was circulated to the friends of both parties. A couple of weeks later the Weils, Louis Bouckaert, Max Krook (a South African astrophysicist), Ralph and myself made a river excursion to Grantchester by punt and canoe to have tea at the Red Lion; there is a photograph of Ralph and myself, with our triumphantly captured lion between us and André Weil looking benevolently on.”

From this and the date of the photograph (May 13th 1939) one can conclude that the marriage-card was drawn up around mid april 1939. As weddings tend to follow their announcement by a couple of months, this contradicts the following passage from Notice sur la Vie et l ‘oeuvre de Nicolas Bourbaki by an unidentified author :



“Nominated as Privat-Dozent at the University of Dorpat in 1913, he (that is, N. Bourbaki) married two years later; a single girl, Betti, married in 1938 to the Lion hunter H. Pétard, was born out of this marriage.”

But then, when was the Bourbaki-Pétard wedding scheduled? Surely, a wedding announcement should provide that information. Here’s the relevant part :



“The trivial isomorphism (aka the sacrament of matrimony) will be given to them by P. Adic, of the Diophantine Order, at the Principal Cohomology of the Universal Variety, the 3 Cartember, year VI, at the usual hour.”

Here’s my guess : the first Bourbaki-meeting took place December 10th 1934. Actually, it was a ‘proto-Bourbaki-meeting’, but nevertheless founding members such as [Jean Delsarte counted 1934 as the first Bourbaki year as is clear from the ‘Remarque’ at the top of his notes of the first meeting : 34+25=59, trying to figure out when the 25-year festivity was going to be held …



Thus, if 1934 is year 1 of the Bourbaki-calender, year VI should be 1939. The notules also give a hint of ‘the usual hour’. In the 1934-1940 period, the Bourbakis met twice a month before the monday-afternoon seminar, at 12 o’clock sharp, the ‘sacred hour’, for a meeting over lunch.

Remains the ‘Cartembre’-puzzle. We know ‘Septembre (7), Novembre (9), Decembre (10)’ so if ‘Cart’ is short for ‘Quatre’ (4), Cartembre might be June. I guess the wedding was scheduled to be held on June 3rd, 1939 at 12h.

It fits with the date the announcement was drawn up and June 3rd, 1939 sure enough was a saturday, the ‘canonical’ day for a wedding. Remains the problem of the wedding place. Suggestions anyone?

6 Comments