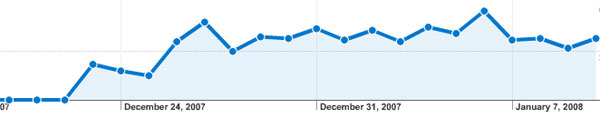
I’ve re-installed the Google analytics plugin on december 22nd, so it is harvesting data for three weeks only. Still, it is an interesting tool to gain insight in the social networking aspect of math-blogging, something I’m still very bad at…
Below the list of all blogs referring at least 10 times over this last three weeks. In brackets are the number of referrals and included are the average time Avg. they spend on this site, as well as the bounce back rate BB. It gives me the opportunity to link back to some of their posts, as a small token of gratitude. I may repeat this in the future, so please keep on linking…
Not Even Wrong (69) : Avg (1.05 min) BB (52.94%)
The most recent post of Peter is an update on the plagiarism scandal on the arXiv.
The n-category cafe (63) : Avg (2.13 min) BB (50%)
The one series I followed at the cafe lately was the Geometric Representation Theory course run by John Baez and James Dolan. They provide downloadable movies as well as notes.
Richard Borcherd’s blog (47) : Avg (1.53 min) BB (53.19%)
It is great to see that Borcherds has taken up blogging again, with a post on the uselessness of set theory.
The Arcadian functor (32) : Avg (3.45 min) BB (34.38 %)
It is clear from the low bounce-back rate and the high average time spend on this site, that Kea’s readers and mine have common interests. Often I feel that Kea and I are talking about the same topics, but that our language is so different, that it is difficult for me to spot the precise connection. I definitely should start (for myself) a translation-project of her M-theory posts.
RupertGee’s iBlog (23) : Avg (6.48 min) BB (34.7 %)
Surprisingly, and contrasting to my previous rant iTouch-people (or at least those coming here from Rupert Gee’s blog) sure take time to read the posts and look for more.
Ars Mathematica (22) : Avg (0:01 min) BB (77,2 %)
Well, the average time and bounce back rate say it all : people coming here from Ars Mathematica are not interested in longer posts…
iTouch Fans Forum (14) : Avg (2:07 min) BB (42.86 %)
Again, better statistics than I would have expected.
Vivatsgasse 7 (13) : Avg (1:51 min) BB (38.46 %)
I hope these guys haven’t completely given up on blogging as it is one of my favourites.
Sixth form mathematics (12) : Avg (1:40 min) BB (25 %)
My few old posts on LaTeXrender still draw referrals…
Strategic Boards (12) : Avg (0:01 min) BB (91.67 %)
People in strategic board games are not really in my game-posts it seems…
The Everything Seminar (11) : Avg (2:04 min) BB (72.73 %)
Greg Muller has been posting a couple of nice posts on chord diagrams, starting here.
Noncommutative Geometry (11) : Avg (3:36 min) BB (27.27 %)
Well, we are interested in the same thing viewed from different angles, so good average times and a low bounce back rate. Maybe, I should make another attempt to have cross-interaction between the two blogs.
7 Comments