In the spring of 2009 I did spend a fortnight dog-sitting in a huge house in the countryside, belonging to my parents-in-law, who both passed away the year before.
That particular day it was raining and thundering heavily. To distract myself from the sombre and spooky atmosphere in the house I began to surf the web looking for material for a new series of blogposts (yes, in those days I was still thinking in ‘series’ of posts…).
Bookmarks for that day tell me that the first picture grasping my attention was Salvador Dali’s Sacrament of the last supper, in particular the depicted partial dodecahedron

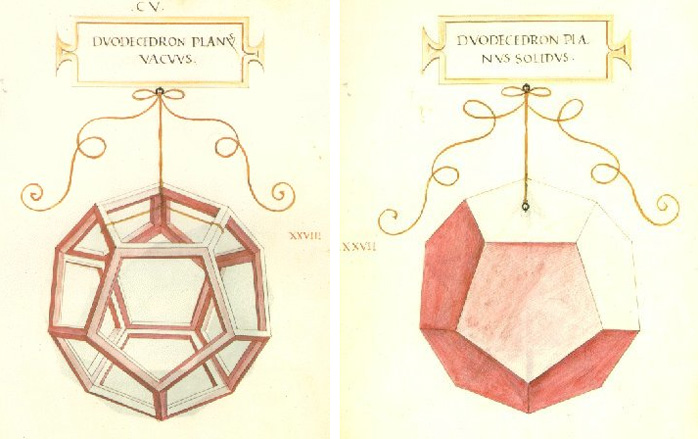
I did compare it with Leonardo’s last supper and in the process stumbled upon Leonardo’s drawings of polyhedra, among which these two dodecahedra

From there it went on and on : the Mystery of the 2nd and 3rd Century Roman Dodecahedron and its posible use ‘casting dodecahedra’ in Tarot Divination Without Tarot Cards or as an astronomical instrument, a text on polyhedra and plagiarism in the renaissance, the history of the truncated icosahedron, a Bosnian pyramid and its stone balls, the sacred geometry of the dodecahedron, mathematics in the Vatican library, and on and on and on…
By noon, I felt I had enough material to post for a couple of weeks on “platonic solids through the ages”.
In between two rain showers, I walked the dog, had a quick lunch, and started writing.
I wanted to approach the topic in chronological order, and as I had done already a quicky on Scottish solids, the first post of the series would have to extend on this picture of five stone balls from the Ashmolean museum (or so it was claimed).
So, I hunted for extra pictures of these stone balls from the Ashmolean, and when comparing the two, clearly something had to be wrong…


It took me a couple of hours to catch up with the scientific literature on these Scottish balls, their cataloguing system and the museums of Scotland and England that house them.
Around 4pm I had compiled a list of all potential dodecahedra and icosahedra Scottish balls: ‘there are only 8 possible candidates for a Scottish dodecahedron (below their catalogue numbers, indicating to the knowledgeable which museum owns them and where they were found)
NMA AS 103 : Aberdeenshire
AS 109 : Aberdeenshire
AS 116 : Aberdeenshire (prob)
AUM 159/9 : Lambhill Farm, Fyvie, Aberdeenshire
Dundee : Dyce, Aberdeenshire
GAGM 55.96 : Aberdeenshire
Montrose = Cast NMA AS 26 : Freelands, Glasterlaw, Angus
Peterhead : Aberdeenshire
The case for a Scottish icosahedron looks even worse. Only two balls have exactly 20 knobs
NMA AS 110 : Aberdeenshire
GAGM 92 106.1. : Countesswells, Aberdeenshire’
About an hour later I’d written the post, clicked the ‘Publish’ button and The Scottish solids hoax, began to live a life of its own!
From the numerous reactions let me single out 3 follow-ups which I believe to be most important.
John McKay and Tom Leinster did some legwork, tracking down resp. photographer and one of the 20 knobs balls.
John Baez gave a talk at an AMS meeting dedicated to the history of mathematics on Who discovered the icosahedron? mentioning my post and extending it by:
“And here is where I did a little research of my own. The library at UC Riverside has a copy of Keith Critchlow’s 1979 book Time Stands Still. In this book, we see the same photo of stones with ribbons that appears in Lawlor’s book – the photo that Atiyah and Suttcliffe use. In Critchlow’s book, these stones are called “a full set of Neolithic ‘Platonic solids'”. He says they were photographed by one Graham Challifour – but he gives no information as to where they came from!
And Critchlow explicitly denies that the Ashmolean has an icosahedral stone! He writes:
… the author has, during the day, handled five of these remarkable objects in the Ashmolean museum…. I was rapt in admiration as I turned over these remarkable stone objects when another was handed to me which I took to be an icosahedron…. On careful scrutiny, after establishing apparent fivefold symmetry on a number of the axes, a count-up of the projections revealed 14! So it was not an icosahedron.”
And now there is even a published paper out!
Bob Lloyd wrote How old are the Platonic solids?, published in BSHM Bulletin: Journal of the British Society for the History of Mathematics. The full article is behind a paywall but Bob graciously send me a copy.

Bob believes the balls in the picture to belong to the Scottish ‘National Museum of Antiquities’ (NMA in the Marshall list), now the National Museum of Scotland (NMS) in Edinburgh.
He believes the third and fourth ball to be two pictures of the same object “recorded as having been discovered in Aberdeenshire” so it should be NMA AS 103 : Aberdeenshire in the above list. (Or, the other one may be NMA AS 26?).
He also attempts to identify the other 3 balls with objects in the NMS-collection. In short, he gives compelling evidence that the picture must have been taking in Edinburg and exists of genuine artifacts.
Perhaps even more important is that he finally puts the case of a Scottish icosahedron to rest. As mentioned above, there are just two candidates NMA AS 110 (Edinburg) and GAGM 92 106.1 (Glasgow). He writes:
“According to the Marshall list, there are only two balls known which have 20K; one of these is at the NMS. Alan Saville, Senior Curator for Earliest Prehistory at this Museum, has provided a photograph which shows that this object is complex, and certainly not a dodecahedron. It could be considered as a modified octahedron, with five large knobs in the usual positions, but with the sixth octahedral position occupied by twelve small knobs, and in addition there are also three small triangles carved at some of the interstices, the three-fold positions of the ‘octahedron’. These make up a total of twenty ‘protrusions’, though the word ‘knobs’ is hard to justify.
The other 20K object is at the Kelvingrove museum in Glasgow. Photographs taken by Tracey Hawkins, assistant curator, show that this also is very far from being a dodecahedron, though this time there are twenty clearly defined knobs of roughly the same size. The shape is somewhat irregular, but two six-sided pyramids can be picked out, and much of the structure, though not all, is deltahedral in form, with sets of three balls at the corners of equilateral triangles.”
So, sadly for John McKay, there is no Scottish icosahedron out there!
One final comment. Both John Baez (in a comment) and Bob Lloyd (in a comment and in his paper) argue that I shouldn’t have used the term “hoax” for something that is merely a ‘matter of sloppy scholarship’.
My apologies.
Given Bob’s evidence that the balls in the picture are genuine artifacts, I have deleted the ‘fabrication or falsification’-phrase in the original post.
Summarizing : the Challifour photograph is not taken at the Ashmolean museum, but at the National Museum of Scotland in Edinburgh and consists of 5 of their artifacts (or 4 if ball 3 and 4 are identical) vaguely resembling cube, tetrahedron, dodecahedron (twice) and octahedron. The fifth Platonic solid, the icosahedron, remains elusive.
Leave a Comment