Skip to content →

neverendingbooks Posts

some smaller steps

Published September 13, 2004 by lievenlb

It
always amazes me how much time I have to waste in trying to get
tech-stuff (such as this weblog) working the way I want. You will barely
notice it but again I spend too much time delving in PHP-scripts,
sometimes with minor success, most of the time almost wrecking this
weblog…

An example : it took me a day to figure out why
this page said there was just 1 visitor online whereas log files showed
otherwise. The PHP-script I used checked this by looking at the
IP-address via _REMOTE_ADDR_ which is perfectly OK on an ordinary
Mac OS 10.3 machine, but _not_ on an OS X-Server! For some reason
it gives as the REMOTE_ADDR just the IP address of the Server (that
is, www.matrix.ua.ac.be in this case) so whoever came by this page got
tagged as 143.129.75.209 and so the script thought there was just one
person around… The trivial way around it is changing every
occurence of REMOTE_ADDR by _HTTP_PC_REMOTE_ADDR_.
Easy enough but it took me a while to figure it out.

Another
example : over the week-end this weblog got a stalker! There were over
100 hits from 38.113.198.9, so whoever that is really liked this site
but didn't have time to read a thing… Again, the standard
solution is to ban the IP-address and most weblog-packages have such a
tool on their admin-page. But whathever I tried and Googled WordPress doesn't seem to have it
on board. There were a few hacks and plugins around claiming to do
something about it but none of them worked! So, I tried more drastic
actions such as editing .htaccess files which I thought would solve
everything (again, no problem under 10.3 but _not_ under
10.3-Server!). Once more, a couple of hours lost trying to figure out
how to get the firewall of a Mac-Server do what I needed. The upshot is
that I know now all dark secrets of the _ipfw_ command, so no
more stalking around this site…

In the process of
grounding my stalker, I decided that I needed better site-stats than my
homemade log-file provided. Fortunetely, this time I picked a package
that worked without too much hassle (one more time I had to make the
REMOTE_ADDR substitution but apart from that all went well). You will
see not too much of the power of this stats-package on the page (apart
from the global counter), I feel that such things are best forgotten
until something strange occurs (like stalkers, spammers and other
weirdos). A nice side-effect though was that for the first time I had a
look at _referring pages_, that is the URL leading to this weblog.
Lots of Google searches (some strange ones) but today there were also a
number of referrals from a Chinese blog. I checked it out and it turned
out to be the brand new Math is Math! Life is Life! weblog…

Another time
consuming thing was getting the BBC-news RSS feeds working in the
sidebar, so that you still get _some_ feel for reality while
being trapped here. I am not yet satisfied with the layout under
Explorer, but then everyone should move on to Safari (so I did give up
trying to work out the PHP-script).

But most time I wasted on
something that so far has left no trace whatsoever here. A plugin that
allows specific posts to be read only by registered users (of a certain
'level', that is WordPress can give users a level from 0 to 10
with specific degrees of freedom). But clearly at the same time I wanted
the rest of the world to have at least some indication of what they were
missing (such as a title with a nice padlock next to it) but so far I
didn't get it working. The only trace of a closed posting would be
in the sidebar-listing of the ten last posts but gives an error message
when an unauthorized user clicks on it. So, still a lot of
headache-sensitive work left to do, but it is about time to get back to
mathematics…

update (febr. 2007) : the
padlock-idea is abandoned.

Leave a Comment

explaining symmetry

Published September 10, 2004 by lievenlb


PseudonomousDaughterTwo learned vector-addition at school and
important formulas such as the _Chasles-Moebius_ equation

$\forall A,B,C \in \mathbb{R}^2~:~\vec{AB}+\vec{BC} = \vec{AC} $

Last evening I helped her a bit with her homework and there was one
problem she could not do immediately (but it was a starred exercise so
you didn't have to do it, but…) :

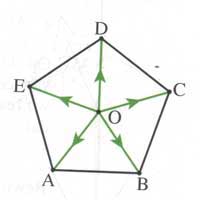
consider a regular pentagon
with center $\vec{0} $. Prove that

$\vec{0A} + \vec{0B} +
\vec{0C} + \vec{0D} + \vec{0E} = \vec{0} $

PD2 : How would
_you_ do this? (with a tone like : I bet even you can't do
it)
Me : Symmetry!
PD2 : Huh?
Me : Rotate the plane
1/5 turn, then $A \mapsto B $, $B \mapsto C $ and so on. So the vector
giving the sum of all five terms must be mapped to itself under this
rotation and the only vector doing this is the zero vector.
PD2 :
That cannot be the solution, you didn't take sums of vectors and all
other exercises did that.
Me : I don't care, it is an elegant
solution, you don't have to compute a thing!

But clearly
she was not convinced and I had to admit there was nothing in her
textbook preparing her for such an argument. I was about to explain that
there was even more symmetry : reflecting along a line through a vertex
giving dihedral symmetry when I saw what the _intended solution_
of the exercise was :

Me : Okay, if you _have_ to do
sums let us try this. Fix a vertex, say A. Then the sum
$\vec{0E}+\vec{0B} $ must lie on the line 0A by the parallellogram-rule
(always good to drop in a word from the textbook to gain some
trust…), similarly the sum $\vec{0C}+\vec{0D} $ must lie on the
line 0A. So you now have to do a sum of three vectors lying on the
line 0A so the result must lie on 0A
PD2 : Yes, and???
Me : But there was nothing special about $A$. I could have started with
B and do the whole argument all over again and then I would get that
the sum is a vector on the line 0B
PD2 : And the only vector
lying on both 0A and 0B is $\vec{0} $
Me : Right! But
all we did now was just redoing the symmetry argument because the line
0A is mapped to 0B
PD2 : Don't you get started on
_that symmetry_ again!

I wonder which of the two
solutions she will sell today as her own. I would love to see the face
of a teacher when a 15yr old says “Clearly that is trivial because
the zero vector is the only one left invariant under
pentagon-symmetry!”

Leave a Comment

cotangent bundles

Published September 9, 2004 by lievenlb

The
previous post in this sequence was [moduli spaces][1]. Why did we spend
time explaining the connection of the quiver
$Q~:~\xymatrix{\vtx{} \ar[rr]^a & & \vtx{} \ar@(ur,dr)^x} $
to moduli spaces of vectorbundles on curves and moduli spaces of linear
control systems? At the start I said we would concentrate on its _double
quiver_ $\tilde{Q}~:~\xymatrix{\vtx{} \ar@/^/[rr]^a && \vtx{}
\ar@(u,ur)^x \ar@(d,dr)_{x^*} \ar@/^/[ll]^{a^*}} $ Clearly,
this already gives away the answer : if the path algebra $C Q$
determines a (non-commutative) manifold $M$, then the path algebra $C
\tilde{Q}$ determines the cotangent bundle of $M$. Recall that for a
commutative manifold $M$, the cotangent bundle is the vectorbundle
having at the point $p \in M$ as fiber the linear dual $(T_p M)^*$ of
the tangent space. So, why do we claim that $C \tilde{Q}$
corresponds to the cotangent bundle of $C Q$? Fix a dimension vector
$\alpha = (m,n)$ then the representation space
$\mathbf{rep}_{\alpha}~Q = M_{n \times m}(C) \oplus M_n(C)$ is just
an affine space so in its point the tangent space is the representation
space itself. To define its linear dual use the non-degeneracy of the
_trace pairings_ $M_{n \times m}(C) \times M_{m \times n}(C)
\rightarrow C~:~(A,B) \mapsto tr(AB)$ $M_n(C) \times M_n(C)
\rightarrow C~:~(C,D) \mapsto tr(CD)$ and therefore the linear dual
$\mathbf{rep}_{\alpha}~Q^* = M_{m \times n}(C) \oplus M_n(C)$ which is
the representation space $\mathbf{rep}_{\alpha}~Q^s$ of the quiver
$Q^s~:~\xymatrix{\vtx{} & & \vtx{} \ar[ll] \ar@(ur,dr)} $
and therefore we have that the cotangent bundle to the representation
space $\mathbf{rep}_{\alpha}~Q$ $T^* \mathbf{rep}_{\alpha}~Q =
\mathbf{rep}_{\alpha}~\tilde{Q}$ Important for us will be that any
cotangent bundle has a natural _symplectic structure_. For a good
introduction to this see the [course notes][2] “Symplectic geometry and
quivers” by [Geert Van de Weyer][3]. As a consequence $C \tilde{Q}$
can be viewed as a non-commutative symplectic manifold with the
symplectic structure determined by the non-commutative 2-form
$\omega = da^* da + dx^* dx$ but before we can define all this we
will have to recall some facts on non-commutative differential forms.
Maybe [next time][4]. For the impatient : have a look at the paper by
Victor Ginzburg [Non-commutative Symplectic Geometry, Quiver varieties,
and Operads][5] or my paper with Raf Bocklandt [Necklace Lie algebras
and noncommutative symplectic geometry][6]. Now that we have a
cotangent bundle of $C Q$ is there also a _tangent bundle_ and does it
again correspond to a new quiver? Well yes, here it is
$\xymatrix{\vtx{} \ar@/^/[rr]^{a+da} \ar@/_/[rr]_{a-da} & & \vtx{}
\ar@(u,ur)^{x+dx} \ar@(d,dr)_{x-dx}} $ and the labeling of the
arrows may help you to work through some sections of the Cuntz-Quillen
paper…

[1]: http://www.neverendingbooks.org/index.php?p=39
[2]: http://www.win.ua.ac.be/~gvdwey/lectures/symplectic_moment.pdf
[3]: http://www.win.ua.ac.be/~gvdwey/
[4]: http://www.neverendingbooks.org/index.php?p=41
[5]: http://www.arxiv.org/abs/math.QA/0005165
[6]: http://www.arxiv.org/abs/math.AG/0010030

2 Comments