neverendingbooks – Page 223

icosahedral group

Published October 21, 2004 by lievenlb

In my geometry 101 course I'm doing the rotation-symmetry groups
of the Platonic solids right now. This goes slightly slower than
expected as it turned out that some secondary schools no longer give a
formal definition of what a group is. So, a lot of time is taken up
explaining permutations and their properties as I want to view the
Platonic groups as subgroups of the permutation groups on the vertices.
To prove that the _tetrahedral group_ is isomorphic to $A_4$ was pretty
straigthforward and I'm half way through proving that the
_octahedral group_ is just $S_4$ (using the duality of the octahedron
with the cube and using the $4$ body diagonals of the cube).
Next
week I have to show that the _icosahedral group_ is isomorphic to $A_5$
which is a lot harder. The usual proof (that is, using the duality
between the icosahedron and the dodecahedron and using the $5$ cubes
contained in the dodecahedron, one for each of the diagonals of a face)
involves too much calculations to do in one hour. An alternative road is
to view the icosahedral group as a subgroup of $S_6$ (using the main
diagonals on the $12$ vertices of the icosahedron) and identifying this
subgroup as $A_5$. A neat exposition of this approach is given by John Baez in his
post Some thoughts on
the number $6$. (He also has another post on the icosahedral group
in his Week 79's
finds in mathematical physics).

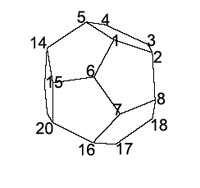But
probably I'll go for an “In Gap we
thrust”-argument. Using the numbers on the left, the rotation by
$72^o$ counter-clockwise in the top face we get the permutation in
$S_{20}$
$(1,2,3,4,5)(6,8,10,12,14)(7,9,11,13,15)(16,17,18,19,20)$
and the
rotation by $72^o$ counterclockwise along the face $(1,2,8,7,8)$ gives
the permutation
$(1,6,7,8,2)(3,5,15,16,9)(4,14,20,17,10)(12,13,19,18,11)$
GAP
calculates that the subgroup $dode$ of $S_{20}$ generated by these two
elements is $60$ (the correct number) and with $IsSimplegroup(dode);$ we
find that this group must be simple. Finally using
$IsomorphismTypeInfoFiniteSimplegroup(dode);$
we get the required
result that the group is indeed isomorphic to $A_5$. The time saved I
can then use to tell something about the classification project of
finite simple groups which might be more inspiring than tedious
calculations…

counting down

Published October 20, 2004 by lievenlb

Clearly the best thing to do for me right now would be to get into
the car and drive a 1000km south to our regular place in France. The
weather should still be good enough to have lunch outside wearing only
a T-shirt, the colours of the leaves will be splendid and the grapes
'a point'. But then we all have resp. work to finish, schools
to attend and courses to teach so all of this will have to be delayed
just a little bit longer…
Some people are more fortunate.
According to her october column, Jeanette Winterson did spend most of
september in France and the daily routine she describes resembles ours
quite well. Mind you, there are differences. Whereas she goes for a
Kir I'll prefer a proper Pastis any time. She drinks the local
white wine, we go for the Rose (weather and supply permitting…)
from the Cave Cooperative 'La Cevenole' in
Rosieres. She only has to cycle 4 kilometers to get into

… a remote village enjoying two bakeries, a
butcher, a greengrocer, a fabulous deli, and a bar.

For us the nearest village having all of this on offer is Joyeuse and it takes 45 minutes by
car along narrow and winding roads to get there (1hr by bicycle to,
2hrs by bicycle back). The more important facts are similar though as
Jeanette Winterson describes in her september column :

It was a good day
to slow down, and now that I am here, I will find a different rhythm,
or rather, re-find the rhythm natural to me.Of course, what’s
important to me may not be important to you, different priorities are
fine, but I think we should make intelligent choices. The fact is that
a lot of what we do isn’t a choice at all, intelligent or stupid, we
just do it passively, and wonder where the day has gone.

How
many days in a life?

And when it’s gone, it’s gone.

I can hear a woodpecker tapping away like me. Maybe that
woodpecker is writing its memoirs. The woodpecker can hear me tapping
too. I am right by its tree, high up, in a top turret room. This
sympathetic Morse code makes me feel part of the life of the tree. It
is easier here to belong to other life forms, other ways of life. I am
aware of all the noises, the scents, the temperature. In the city, we
spend a lot of energy shutting life out – the noise, the aggression,
the crowded feel. It is such a relief to let life in, and even as I
write those words, I wonder how bonkers we are, making a life that is
anti-life; a life where life has to be shut out.

a cosmic Galois group

Published October 6, 2004 by lievenlb

Are
there hidden relations between mathematical and physical constants such
as

$\frac{e^2}{4 \pi \epsilon_0 h c} \sim \frac{1}{137} $

or are these numerical relations mere accidents? A couple of years
ago, Pierre Cartier proposed in his paper A mad day’s work : from Grothendieck to Connes and
Kontsevich : the evolution of concepts of space and symmetry that
there are many reasons to believe in a cosmic Galois group acting on the
fundamental constants of physical theories and responsible for relations
such as the one above.

The Euler-Zagier numbers are infinite
sums over $n_1 > n_2 > ! > n_r \geq 1 $ of the form

$\zeta(k_1,\dots,k_r) = \sum n_1^{-k_1} \dots n_r^{-k_r} $

and there are polynomial relations with rational coefficients between
these such as the product relation

$\zeta(a)\zeta(b)=\zeta(a+b)+\zeta(a,b)+\zeta(b,a) $

It is
conjectured that all polynomial relations among Euler-Zagier numbers are
consequences of these product relations and similar explicitly known
formulas. A consequence of this conjecture would be that
$\zeta(3),\zeta(5),\dots $ are all trancendental!

Drinfeld
introduced the Grothendieck-Teichmuller group-scheme over $\mathbb{Q} $
whose Lie algebra $\mathfrak{grt}_1 $ is conjectured to be the free Lie
algebra on infinitely many generators which correspond in a natural way
to the numbers $\zeta(3),\zeta(5),\dots $. The Grothendieck-Teichmuller
group itself plays the role of the Galois group for the Euler-Zagier
numbers as it is conjectured to act by automorphisms on the graded
$\mathbb{Q} $-algebra whose degree $d $-term are the linear combinations
of the numbers $\zeta(k_1,\dots,k_r) $ with rational coefficients and
such that $k_1+\dots+k_r=d $.

The Grothendieck-Teichmuller
group also appears mysteriously in non-commutative geometry. For
example, the set of all Kontsevich deformation quantizations has a
symmetry group which Kontsevich conjectures to be isomorphic to the
Grothendieck-Teichmuller group. See section 4 of his paper Operads and motives in
deformation quantzation for more details.

It also appears
in the renormalization results of Alain Connes and Dirk Kreimer. A very
readable introduction to this is given by Alain Connes himself in Symmetries Galoisiennes
et renormalisation. Perhaps the latest news on Cartier’s dream of a
cosmic Galois group is the paper by Alain Connes and Matilde Marcolli posted
last month on the arXiv : Renormalization and
motivic Galois theory. A good web-page on all of this, including
references, can be found here.

neverendingbooks Posts

icosahedral group

counting down

a cosmic Galois group