probably, you’ve never seen the dedication in my one quiver to rule them
all paper on the arXiv

unfortunately, i’ve not much to add to this, even 3 years later…
Leave a CommentThree years ago I did spend three weeks next to my Canonscan, painstakingly scanning all individual pages of every preprint I ever wrote. Next, I converted every page to PDF, resized it (in order to control the size) and bundled them into PDF-files. A typical preprint would take me roughly three quarters of an hour and the final result was mediocre. For example, here a blown-up sample from the original 1992 ‘Moduli
spaces of right ideals of the Weyl algebra’ -preprint, resulting in a 1.7Mb PDF-file
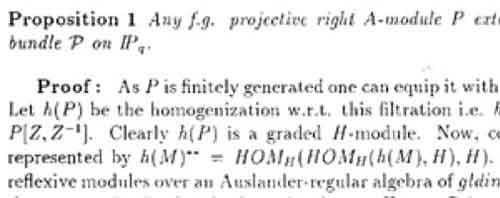
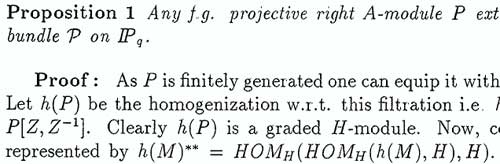
Recentlty, the department bought a Ricoh-copier which makes scanning a lot more fun. To scan a preprint at 300dpi and convert it into a single PDF-file takes under a minute (actually, downloading the file using a web-interface takes longer…). For this particular preprint, the resulting PDF-file took up 1.2Mb and looks a lot nicer

Still, 1.2Mb is a huge file but converting it to a DjVu-file (DjVu=deja vu) using the handy Any2DjVu Service gives us a mere 236Kb file which comes a lot closer to the filesize of a PDFLaTeX-file and the output is still very legible
So, I decided to rescan my entire life at 300dpi and convert it into DjVu. Next, I got the MOPP-package (MOPP = My Online Publications Page) working using the instructions from this page and some obvious MacOSX-modifications (if I can do it, so can you but perhaps I’ll write up the details in another post, just to remind myself). You can see the result at my homepage. I’ll update the latter one regularly (there are still some preprints missing, as are all my courses etc. and cross-references) and only afterwards I’ll update my homepage again. So far there is 250Mb to download (including all versions of the noncommutative geometry@n book, including the published ones…) so this should keep you busy for a while…
Leave a Comment