Over the last days I’ve been staring at the Bost-Connes algebra to find a ringtheoretic way into it. Ive had some chats about it with the resident graded-guru but all we came up with so far is that it seems to be an extension of Fred’s definition of a ‘crystalline’ graded algebra. Knowing that several excellent ringtheorists keep an eye on my stumblings here, let me launch an appeal for help :
What is the most elegant ringtheoretic framework in which the Bost-Connes Hecke algebra is a motivating example?
Let us review what we know so far and extend upon it with a couple of observations that may (or may not) be helpful to you. The algebra $\mathcal{H} $ is the algebra of $\mathbb{Q} $-valued functions (under the convolution product) on the double coset-space $\Gamma_0 \backslash \Gamma / \Gamma_0 $ where
$\Gamma = { \begin{bmatrix} 1 & b \\ 0 & a \end{bmatrix}~:~a,b \in \mathbb{Q}, a > 0 } $ and $\Gamma_0 = { \begin{bmatrix} 1 & n \\ 0 & 1 \end{bmatrix}~:~n \in \mathbb{N}_+ } $
We have seen that a $\mathbb{Q} $-basis is given by the characteristic functions $X_{\gamma} $ (that is, such that $X_{\gamma}(\gamma’) = \delta_{\gamma,\gamma’} $) with $\gamma $ a rational point represented by the couple $~(a,b) $ (the entries in the matrix definition of a representant of $\gamma $ in $\Gamma $) lying in the fractal comb
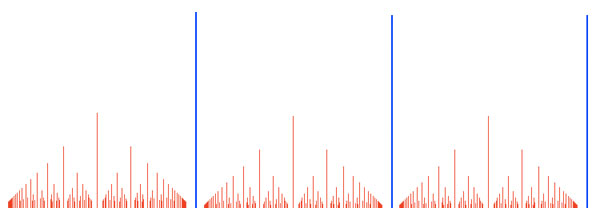
defined by the rule that $b < \frac{1}{n} $ if $a = \frac{m}{n} $ with $m,n \in \mathbb{N}, (m,n)=1 $. Last time we have seen that the algebra $\mathcal{H} $ is generated as a $\mathbb{Q} $-algebra by the following elements (changing notation)
$\begin{cases}X_m=X_{\alpha_m} & \text{with } \alpha_m = \begin{bmatrix} 1 & 0 \\ 0 & m \end{bmatrix}~\forall m \in \mathbb{N}_+ \\
X_n^*=X_{\beta_n} & \text{with } \beta_n = \begin{bmatrix} 1 & 0 \\ 0 & \frac{1}{n} \end{bmatrix}~\forall n \in \mathbb{N}_+ \\
Y_{\gamma} = X_{\gamma} & \text{with } \gamma = \begin{bmatrix} 1 & \gamma \\ 0 & 1 \end{bmatrix}~\forall \lambda \in \mathbb{Q}/\mathbb{Z} \end{cases} $
Using the tricks of last time (that is, figuring out what functions convolution products represent, knowing all double-cosets) it is not too difficult to prove the defining relations among these generators to be the following (( if someone wants the details, tell me and I’ll include a ‘technical post’ or consult the Bost-Connes original paper but note that this scanned version needs 26.8Mb ))
(1) : $X_n^* X_n = 1, \forall n \in \mathbb{N}_+$
(2) : $X_n X_m = X_{nm}, \forall m,n \in \mathbb{N}_+$
(3) : $X_n X_m^* = X_m^* X_n, \text{whenever } (m,n)=1$
(4) : $Y_{\gamma} Y_{\mu} = Y_{\gamma+\mu}, \forall \gamma,mu \in \mathbb{Q}/\mathbb{Z}$
(5) : $Y_{\gamma}X_n = X_n Y_{n \gamma},~\forall n \in \mathbb{N}_+, \gamma \in \mathbb{Q}/\mathbb{Z}$
(6) : $X_n Y_{\lambda} X_n^* = \frac{1}{n} \sum_{n \delta = \gamma} Y_{\delta},~\forall n \in \mathbb{N}_+, \gamma \in \mathbb{Q}/\mathbb{Z}$
Simple as these equations may seem, they bring us into rather uncharted ringtheoretic territories. Here a few fairly obvious ringtheoretic ingredients of the Bost-Connes Hecke algebra $\mathcal{H} $
the group-algebra of $\mathbb{Q}/\mathbb{Z} $
The equations (4) can be rephrased by saying that the subalgebra generated by the $Y_{\gamma} $ is the rational groupalgebra $\mathbb{Q}[\mathbb{Q}/\mathbb{Z}] $ of the (additive) group $\mathbb{Q}/\mathbb{Z} $. Note however that $\mathbb{Q}/\mathbb{Z} $ is a torsion group (that is, for all $\gamma = \frac{m}{n} $ we have that $n.\gamma = (\gamma+\gamma+ \ldots + \gamma) = 0 $). Hence, the groupalgebra has LOTS of zero-divisors. In fact, this group-algebra doesn’t have any good ringtheoretic properties except for the fact that it can be realized as a limit of finite groupalgebras (semi-simple algebras)
$\mathbb{Q}[\mathbb{Q}/\mathbb{Z}] = \underset{\rightarrow}{lim}~\mathbb{Q}[\mathbb{Z}/n \mathbb{Z}] $
and hence is a quasi-free (or formally smooth) algebra, BUT far from being finitely generated…
the grading group $\mathbb{Q}^+_{\times} $
The multiplicative group of all positive rational numbers $\mathbb{Q}^+_{\times} $ is a torsion-free Abelian ordered group and it follows from the above defining relations that $\mathcal{H} $ is graded by this group if we give
$deg(Y_{\gamma})=1,~deg(X_m)=m,~deg(X_n^*) = \frac{1}{n} $
Now, graded algebras have been studied extensively in case the grading group is torsion-free abelian ordered AND finitely generated, HOWEVER $\mathbb{Q}^+_{\times} $ is infinitely generated and not much is known about such graded algebras. Still, the ordering should allow us to use some tricks such as taking leading coefficients etc.
the endomorphisms of $\mathbb{Q}[\mathbb{Q}/\mathbb{Z}] $
We would like to view the equations (5) and (6) (the latter after multiplying both sides on the left with $X_n^* $ and using (1)) as saying that $X_n $ and $X_n^* $ are normalizing elements. Unfortunately, the algebra morphisms they induce on the group algebra $\mathbb{Q}[\mathbb{Q}/\mathbb{Z}] $ are NOT isomorphisms, BUT endomorphisms. One source of algebra morphisms on the group-algebra comes from group-morphisms from $\mathbb{Q}/\mathbb{Z} $ to itself. Now, it is known that
$Hom_{grp}(\mathbb{Q}/\mathbb{Z},\mathbb{Q}/\mathbb{Z}) \simeq \hat{\mathbb{Z}} $, the profinite completion of $\mathbb{Z} $. A class of group-morphisms of interest to us are the maps given by multiplication by n on $\mathbb{Q}/\mathbb{Z} $. Observe that these maps are epimorphisms with a cyclic order n kernel. On the group-algebra level they give us the epimorphisms
$\mathbb{Q}[\mathbb{Q}/\mathbb{Z}] \longrightarrow^{\phi_n} \mathbb{Q}[\mathbb{Q}/\mathbb{Z}] $ such that $\phi_n(Y_{\lambda}) = Y_{n \lambda} $ whence equation (5) can be rewritten as $Y_{\lambda} X_n = X_n \phi_n(Y_{\lambda}) $, which looks good until you think that $\phi_n $ is not an automorphism…
There are even other (non-unital) algebra endomorphisms such as the map $\mathbb{Q}[\mathbb{Q}/\mathbb{Z}] \rightarrow^{\psi_n} R_n $ defined by $\psi_n(Y_{\lambda}) = \frac{1}{n}(Y_{\frac{\lambda}{n}} + Y_{\frac{\lambda + 1}{n}} + \ldots + Y_{\frac{\lambda + n-1}{n}}) $ and then, we can rewrite equation (6) as $Y_{\lambda} X_n^* = X_n^* \psi_n(Y_{\lambda}) $, but again, note that $\psi_n $ is NOT an automorphism.
almost strongly graded, but not quite…
Recall from last time that the characteristic function $X_a $ for any double-coset-class $a \in \Gamma_0 \backslash \Gamma / \Gamma_0 $ represented by the matrix $a=\begin{bmatrix} 1 & \lambda \\ 0 & \frac{m}{n} \end{bmatrix} $ could be written in the Hecke algebra as $X_a = n X_m Y_{n \lambda} X_n^* = n Y_{\lambda} X_m X_n^* $. That is, we can write the Bost-Connes Hecke algebra as
$\mathcal{H} = \oplus_{\frac{m}{n} \in \mathbb{Q}^+_{\times}}~\mathbb{Q}[\mathbb{Q}/\mathbb{Z}] X_mX_n^* $
Hence, if only the morphisms $\phi_n $ and $\psi_m $ would be automorphisms, this would say that $\mathcal{H} $ is a strongly $\mathbb{Q}^+_{\times} $-algebra with part of degree one the groupalgebra of $\mathbb{Q}/\mathbb{Z} $.
However, they are not. But there is an extension of the notion of strongly graded algebras which Fred has dubbed crystalline graded algebras in which it is sufficient that the algebra maps are all epimorphisms. (maybe I’ll post about these algebras, another time). However, this is not the case for the $\psi_m $…
So, what is the most elegant ringtheoretic framework in which the algebra $\mathcal{H} $ fits??? Surely, you can do better than generalized crystalline graded algebra…
5 Comments On
On