Category: books

let’s spend 3K on (math)books

Published December 8, 2016 by lievenlb

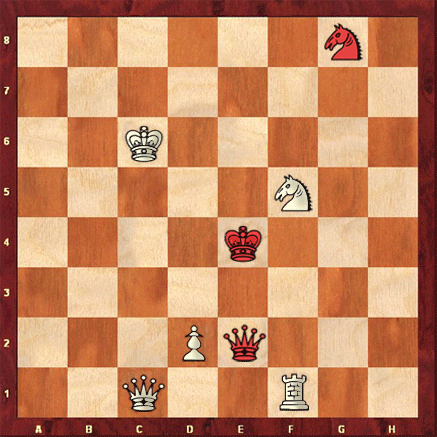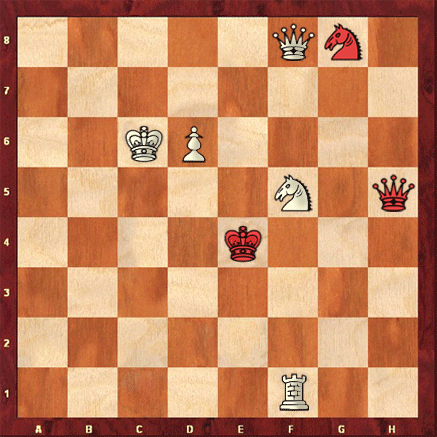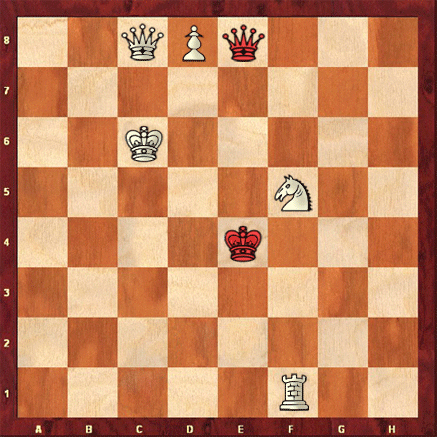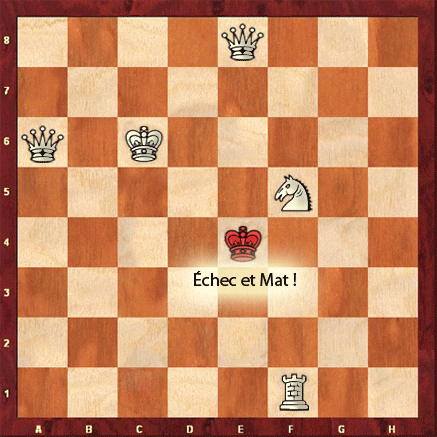
Santa gave me 3000 Euros to spend on books. One downside: I have to give him my wish-list before monday. So, I’d better get started. Clearly, any further suggestions you might have will be much appreciated, either in the comments below or more directly via email.

Today I’ll focus on my own interests: algebraic geometry, non-commutative geometry and representation theory. I do own a fair amount of books already which accounts for the obvious omissions in the lists below (such as Hartshorne, Mumford or Eisenbud-Harris in AG, Fulton-Harris in RT or the ‘bibles’ in NCG).

[section_title text=”Algebraic geometry”]

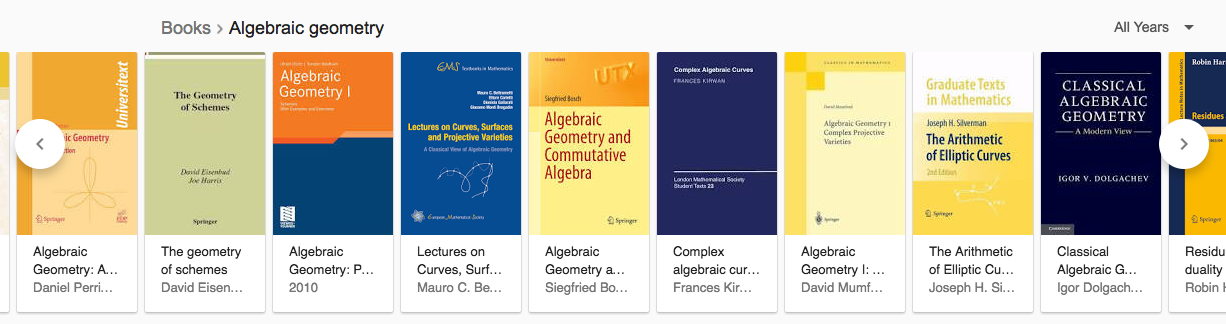
Here, I base myself on (and use quotes from) the excellent answer by Javier Alvarez to the MathOverflow post Best Algebraic Geometry text book? (other than Hartshorne).

In no particular order:

Lectures on Curves, Surfaces and Projective Varieties by Ettore Carletti, Dionisio Gallarati, and Giacomo Monti Bragadin and Mauro C. Beltrametti.
“which starts from the very beginning with a classical geometric style. Very complete (proves Riemann-Roch for curves in an easy language) and concrete in classic constructions needed to understand the reasons about why things are done the way they are in advanced purely algebraic books. There are very few books like this and they should be a must to start learning the subject. (Check out Dolgachev’s review.)”

A Royal Road to Algebraic Geometry by Audun Holme. “This new title is wonderful: it starts by introducing algebraic affine and projective curves and varieties and builds the theory up in the first half of the book as the perfect introduction to Hartshorne’s chapter I. The second half then jumps into a categorical introduction to schemes, bits of cohomology and even glimpses of intersection theory.”

Liu Qing – “Algebraic Geometry and Arithmetic Curves”. “It is a very complete book even introducing some needed commutative algebra and preparing the reader to learn arithmetic geometry like Mordell’s conjecture, Faltings’ or even Fermat-Wiles Theorem.”

Görtz; Wedhorn – Algebraic Geometry I, Schemes with Examples and Exercises. labeled ‘the best on schemes’ by Alvarez. “Tons of stuff on schemes; more complete than Mumford’s Red Book. It does a great job complementing Hartshorne’s treatment of schemes, above all because of the more solvable exercises.”

Kollár – Lectures on Resolution of Singularities. “Great exposition, useful contents and examples on topics one has to deal with sooner or later.”

Kollár; Mori – Birational Geometry of Algebraic Varieties. “Considered as harder to learn from by some students, it has become the standard reference on birational geometry.”

And further, as a follow-up on their previous book on the computational side of AG:

Using Algebraic Geometry by Cox, Little and O’Shea.

[section_title text=”Non-commutative geometry”]

Noncommutative Geometry and Particle Physics by Walter van Suijlekom. Blurb: “This book provides an introduction to noncommutative geometry and presents a number of its recent applications to particle physics. It is intended for graduate students in mathematics/theoretical physics who are new to the field of noncommutative geometry, as well as for researchers in mathematics/theoretical physics with an interest in the physical applications of noncommutative geometry. In the first part, we introduce the main concepts and techniques by studying finite noncommutative spaces, providing a “light” approach to noncommutative geometry. We then proceed with the general framework by defining and analyzing noncommutative spin manifolds and deriving some main results on them, such as the local index formula. In the second part, we show how noncommutative spin manifolds naturally give rise to gauge theories, applying this principle to specific examples. We subsequently geometrically derive abelian and non-abelian Yang-Mills gauge theories, and eventually the full Standard Model of particle physics, and conclude by explaining how noncommutative geometry might indicate how to proceed beyond the Standard Model.”

An Invitation To Noncommutative Geometry by Matilde Marcolli. Blurb: “This is the first existing volume that collects lectures on this important and fast developing subject in mathematics. The lectures are given by leading experts in the field and the range of topics is kept as broad as possible by including both the algebraic and the differential aspects of noncommutative geometry as well as recent applications to theoretical physics and number theory.”

Noncommutative Geometry and Physics: Renormalisation, Motives, Index Theory. Blurb: “This collection of expository articles grew out of the workshop “Number Theory and Physics” held in March 2009 at The Erwin Schrödinger International Institute for Mathematical Physics, Vienna. The common theme of the articles is the influence of ideas from noncommutative geometry (NCG) on subjects ranging from number theory to Lie algebras, index theory, and mathematical physics. Matilde Marcolli’s article gives a survey of relevant aspects of NCG in number theory, building on an introduction to motives for beginners by Jorge Plazas and Sujatha Ramdorai.”

Feynman Motives by Matilde Marcolli. Blurb: “This book presents recent and ongoing research work aimed at understanding the mysterious relation between the computations of Feynman integrals in perturbative quantum field theory and the theory of motives of algebraic varieties and their periods. One of the main questions in the field is understanding when the residues of Feynman integrals in perturbative quantum field theory evaluate to periods of mixed Tate motives.” But then, check out Matilde’s recent FaceBook status-update.

[section_title text=”Representation theory”]

An Introduction to the Langlands Program by J. Bernstein (editor). Blurb: “This book presents a broad, user-friendly introduction to the Langlands program, that is, the theory of automorphic forms and its connection with the theory of L-functions and other fields of mathematics. Each of the twelve chapters focuses on a particular topic devoted to special cases of the program. The book is suitable for graduate students and researchers.”

Representation Theory of Finite Groups: An Introductory Approach by Benjamin Steinberg.

Representation Theory of Finite Monoids by Benjamin Steinberg. Blurb: “This first text on the subject provides a comprehensive introduction to the representation theory of finite monoids. Carefully worked examples and exercises provide the bells and whistles for graduate accessibility, bringing a broad range of advanced readers to the forefront of research in the area. Highlights of the text include applications to probability theory, symbolic dynamics, and automata theory. Comfort with module theory, a familiarity with ordinary group representation theory, and the basics of Wedderburn theory, are prerequisites for advanced graduate level study.”

How am I doing? 914 dollars…

Way to go, same exercise tomorrow. Again, suggestions/warnings welcome!

human-, computer- and fairy-chess

Published December 1, 2016 by lievenlb

It was fun following the second game last night in real time. Carlsen got a winning endgame with two bishops against a rook, but blundered with 62. Bg4?? (winning was Kf7), resulting in stalemate.

There was this hilarious message around move 60:

“The computer has just announced that white mates in 31 moves. Of course, the only two people in the building who don’t benefit from that knowledge are behind the pieces.”

[section_title text=”Alice’s game from ‘Through the Looking-Glass'”]

The position below comes from the preface of Lewis Carroll’s Through the Looking-Glass

The old notation for files is used:

a = QR (queen’s side rook)
b = QKt (queen’s side knight)
c = QB (queen’s side bishop)
d = Q (queen)
e = K (king)
f = KB (king’s side bishop)
g = KKt (king’s side knight)
h = KR (king’s side rook)

Further, the row-number depends on whose playing (they both count starting from their own side). Here’s an animated version of the game:

And a very strange game it is.

White makes consecutive moves, which is allowed in some versions of fairy chess.

And, as the late Martin Gardner explains in his book The Annotated Alice:

“The most serious violation of chess rules occurs near the end of the
problem, when the White King is placed in check by the Red Queen without
either side taking account of the fact. “Hardly a move has a sane purpose,
from the point of view of chess,” writes Mr. Madan. It is true that both sides
play an exceedingly careless game, but what else could one expect from the
mad creatures behind the mirror? At two points the White Queen passes up
a chance to checkmate and on another occasion she flees from the Red
Knight when she could have captured him. Both oversights, however, are in
keeping with her absent-mindedness.”

In fact, the whole game reflects the book’s story (Alice is the white pawn travelling to the other side of the board), with book-pages associated to the positions listed on the left. Martin Gardner on this:

“Considering the staggering difficulties involved in dovetailing a chess
game with an amusing nonsense fantasy, Carroll does a remarkable job. At
no time, for example, does Alice exchange words with a piece that is not
then on a square alongside her own. Queens bustle about doing things while
their husbands remain relatively fixed and impotent, just as in actual chess
games. The White Knight’s eccentricities fit admirably the eccentric way in
which Knights move; even the tendency of the Knights to fall off their
horses, on one side or the other, suggests the knight’s move, which is two
squares in one direction followed by one square to the right or left. In order
to assist the reader in integrating the chess moves with the story, each move
will be noted in the text at the precise point where it occurs.”

The starting position is in itself an easy chess-problem: white mates in 3, as explained by Gardner:

” It is amusing to note that it is the Red Queen who persuades Alice to advance along her file to the eighth square. The Queen is protecting herself with this advice, for white has at the outset an easy, though inelegant, checkmate in three moves.
The White Knight first checks at KKt.3. If the Red King moves to either Q6
or Q5, white can mate with the Queen at QB3. The only alternative is for
the Red King to move to K4. The White Queen then checks on QB5,
forcing the Red King to K3. The Queen then mates on Q6. This calls, of
course, for an alertness of mind not possessed by either the Knight or
Queen. ”

NaNoWriMo (3)

Published November 30, 2016 by lievenlb

In 2001, Eugenia Cheng gave an interesting after-dinner talk Mathematics and Lego: the untold story. In it she compared math research to fooling around with lego. A quote:

“Lego: the universal toy. Enjoyed by people of all ages all over the place. The idea is simple and brilliant. Start with some basic blocks that can be joined together. Add creativity, imagination and a bit of ingenuity. Build anything.

Mathematics is exactly the same. We start with some basic building blocks and ways of joining them together. And then we use creativity, and, yes, imagination and certainly ingenuity, and try to build anything.”

She then goes on to explain category theory, higher dimensional topology, and the process of generalisation in mathematics, whole the time using lego as an analogy. But, she doesn’t get into the mathematics of lego, perhaps because the talk was aimed at students and researchers of all levels and all disciplines.

There are plenty of sites promoting lego in the teaching of elementary mathematics, here’s just one link-list-page: “27 Fantastic LEGO Math Learning Activities for All Ages”. I’m afraid ‘all ages’ here means: under 10…

$lego-math-teaching-children-alycia-zimmerman-fb__700-png$

Can one do better?

Everyone knows how to play with lego, which shapes you can build, and which shapes are simply impossible.

Can one tap into this subconscious geometric understanding to explain more advanced ideas such as symmetry, topological spaces, sheaves, categories, perhaps even topos theory… ?

Let’s continue our

[section_title text=”imaginary iterview”]

Question: What will be the opening scene of your book?

Alice posts a question on Lego-stackexchenge. She wants help to get hold of all imaginary lego shapes, including shapes impossible to construct in three-dimensional space, such as gluing two shapes over some internal common sub-shape, or Escher like constructions, and so on.

Question: And does she get help?

At first she only gets snide remarks, style: “brush off your French and wade through SGA4”.

Then, she’s advised to buy a large notebook and jot down whatever she can tell about shapes that one can construct.

If you think about this, you’ll soon figure out that you can only add new bricks along the upper or lower bricks of the shape. You may call these the boundary of the shape, and soon you’ll be doing topology, and forming coproducts.

These ‘legal’ lego shapes form what some of us would call a category, with a morphism from $A$ to $B$ for each different way one can embed shape $A$ into $B$.

Of course, one shouldn’t use this terminology, but rather speak of different instruction-manuals to get $B$ out of $A$ (the morphisms), stapling two sets of instructions together (the compositions), and the empty instruction-sheet (the identity morphism).

Question: But can one get to the essence of categorical results in this way?

Take Yoneda’s lemma. In the case of lego shapes it says that you know a shape once you know all morphisms into it from whatever shape.

For any coloured brick you’re given the number of ways this brick sits in that shape, so you know all the shape’s bricks. Then you may try for combination of two bricks, and so on. It sure looks like you’re going to be able to reconstruct the shape from all this info, but this quickly get rather messy.

But then, someone tells you the key argument in Yoneda’s proof: you only have to look for the shape to which the identity morphism is assigned. Bingo!

Question: Wasn’t your Alice interested in the ‘illegal’ or imaginary shapes?

Once you get to Yoneda, the rest follows routinely. You define presheaves on this category, figure out that you get a whole bunch of undesirable things, bring in Grothendieck topologies to be the policing agency weeding out that mess, and keep only the sheaves, which are exactly the desired imaginary shapes.

Question: Your book’s title is ‘Primes and other imaginary shapes’. How do you get from Lego shapes to prime numbers?

By the standard Gödelian trick: assign a prime number to each primitive coloured brick, and to a shape the product of the brick-primes.

That number is a sort of code of the shape. Shapes sharing the same code are made up from the same set of bricks.

Take the set of all strictly positive natural numbers partially ordered by divisibility, then this code is a functor from Lego shapes to numbers. If we extend this to imaginary shapes, we’ll rapidly end up at Connes’ arithmetic site, supernatural numbers, adeles and the recent realisation that the set of all prime numbers does have a geometric shape, but one with infinitely many dimensions.

Not sure yet how to include all of this, but hey, early days.

Question: So, shall we continue this interview at a later date?

No way, I’d better start writing.